Give an Example of a Function F Such That F is Everywhere Continous but Not Uniformly Continuous
Function example? Continuous everywhere, differentiable nowhere
Solution 1
The Weierstrass function mentioned in Jesse Madnick's answer is the standard example, but I think this example is slightly misleading. The fact that it is constantly presented as the standard example may suggest that such examples are rare and must be constructed in a certain way. Actually such examples are extremely common; in an appropriate sense, the "generic" continuous function is nowhere differentiable.
To my mind, the point of the Weierstrass function as an example is really to hammer in the following points:
- The uniform limit of continuous functions must be continuous, but
- The uniform limit of differentiable functions need not be differentiable.
However, if $f_n(x)$ is a uniformly convergent sequence of differentiable functions such that the derivatives $f_n'(x)$ also converge uniformly, then the uniform limit $f(x)$ is differentiable, and $f'(x)$ is the uniform limit of the functions $f_n'(x)$. So what fails in the example of the Weierstrass function is that the derivatives do not even come close to converging uniformly.
Solution 2
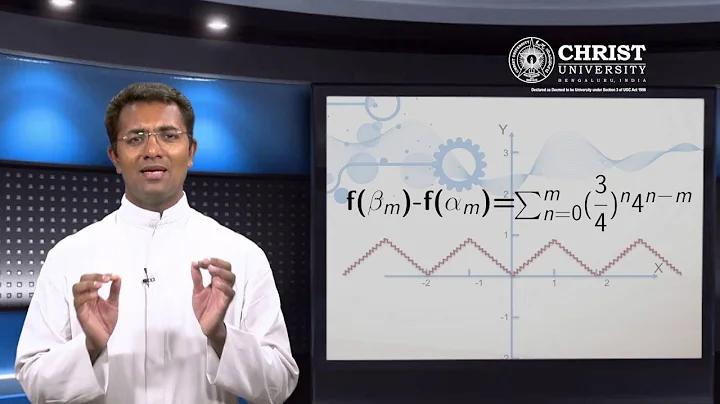
Another popular example is what I know as Takagi's Function.
It is somehow different from the Weierstrass Function in that it is not constructed as a uniform limit of differentiable functions. However, it is a uniform limit of continuous functions in a way that the points of non-differentiability populate the "whole interval" (if that point of view makes any sense...).
Solution 3
A very famous example - and by far the most important when it comes to practical applications (finance: option pricing!) - is the Wiener process.
Related videos on Youtube
19 : 02
Real Continuous Nowhere Differentiable function. Real analysis
32 : 34
continuous everywhere but differentiable nowhere | Analysis | BSc Mathematics
53 : 42
Mod-09 Lec-50 Construction of Everywhere Continuous Nowhere Differentiable Function
07 : 54
Everywhere Continuous Nowhere differentiable function
14 : 32
Continuous Functions that are Differentiable Nowhere
Comments
-
Possible Duplicate:
Are Continuous Functions Always Differentiable?If such a function exists, can anyone give an example of a function $f(x) : \mathbb{R} \longrightarrow \mathbb{R}$ that is continuous for all $x \in \mathbb{R}$ but differentiable nowhere?
-
I cut and pasted the title of your question into google, and the first hit I got was the wikipedia article for the Weierstrass function. So it seems like you didn't put any effort into finding the answer to your question.
-
Even though the question is not the exact same, I am voting to close. In any case, I am pretty sure there are other candidates for dupe, so if anyone can find it...
-
-
I don't really think the Weierstrass function is "slightly misleading". Sure, there are plenty of other examples, but that's nothing against this example...
-
+1: I prefer this to Weierstrass's function since it is much more elementary (and maybe because I've sweated a lot when I had to prove its properties for the first time).
-
@Pete: I may be misremembering this, but there is a math.SE question entitled something like "are there any functions which are continuous everywhere and differentiable nowhere besides the Weierstrass function"? My issue is not with the example but with the tendency to cite the example as if that were the last thing to say about the subject.
-
this i think is good because its something thats easy to remember and draw pictures for (if giving it as an example to someone)
-
Right, I agree wholeheartedly with your last sentence. Don't blame it on Weierstrass!
-
So how would one make the point that such functions are in fact very common, and avoid the suggestion that such examples are rare, while simultaneously providing a tangible example or examples?
-
@mike4ty4 Actually, the set of nowhere differentiable functions is dense in the set of continuous functions (with respect to the uniform topology). See also this answer: math.stackexchange.com/a/165731/3148
Recents
Related
Source: https://9to5science.com/function-example-continuous-everywhere-differentiable-nowhere
0 Response to "Give an Example of a Function F Such That F is Everywhere Continous but Not Uniformly Continuous"
Post a Comment